Investigating P-Delta instabilities in RISA-3D (or RISA-2D) can be difficult. The first step is usually to solve the model without the P-Delta effect included to see if there are any obvious deflection problems that could cause issues with a P-Delta analysis. But, what can be done when this doesn’t yield any obvious issues?
It helps to think of a P-Delta failure as an elastic buckling of your model. It may be a localized buckling of one member, or it may be a general buckling of the frame. The challenging part to understanding the failure is to determine what the buckled shape of the structure looks like.
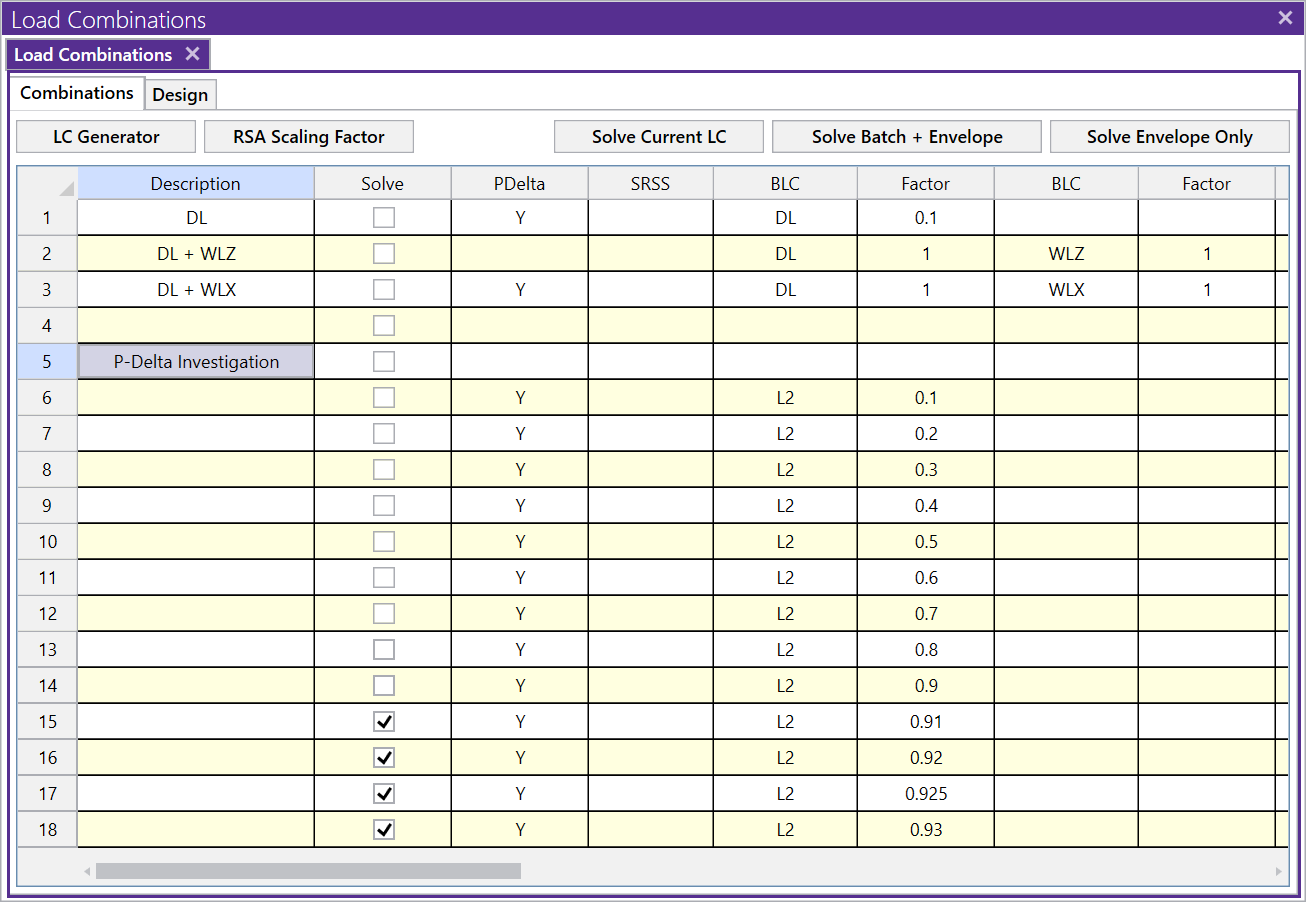
Since the program doesn’t give any results for a P-Delta divergence, the user needs to reduce the loading to the point where the structure is very close to buckling. Take a look at the screen shot below.
Of the normal Load Combinations, LC#2 is the one which diverges for P-Delta. Therefore, we have created a number of load combinations to test this P-Delta failure. These load combination apply a fraction of the load (from 10% to 93%) from LC#2. In this way, we are reducing the load to a point where the structure is very close to buckling, but not quite there.
The deflected shape for LC#17 (which applies 92.5% of the load from LC#2) yields the following images which show nicely what the structure looks like as it begins to buckle.
From these pictures, it becomes clear that the top chord of the truss is buckling out of plane. If there is any doubt about what the buckling is, then we would run LC#17 with and without P-Delta and compare the joint deflections to see which deflections are receiving the greatest amplification due to 2nd order effects.
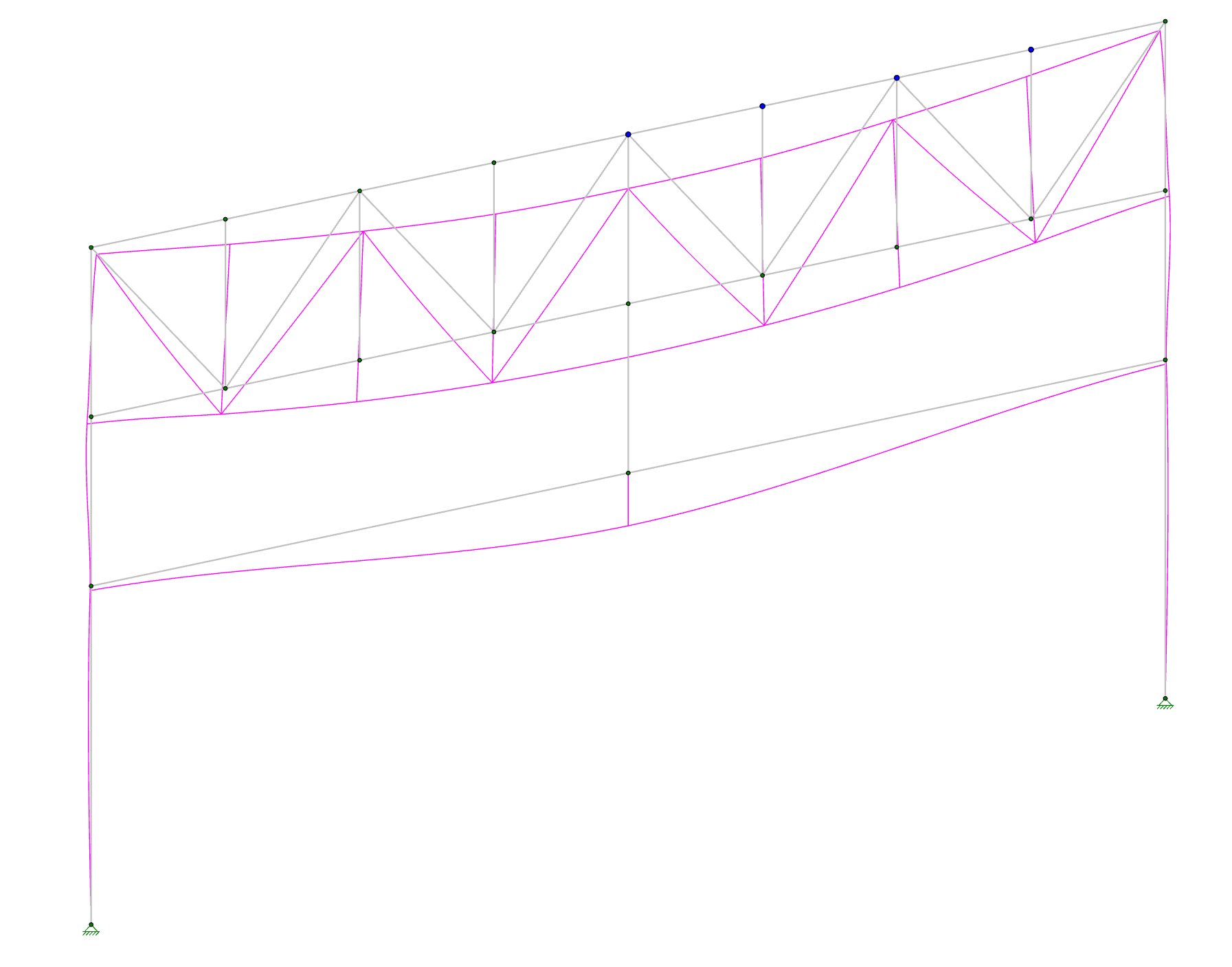
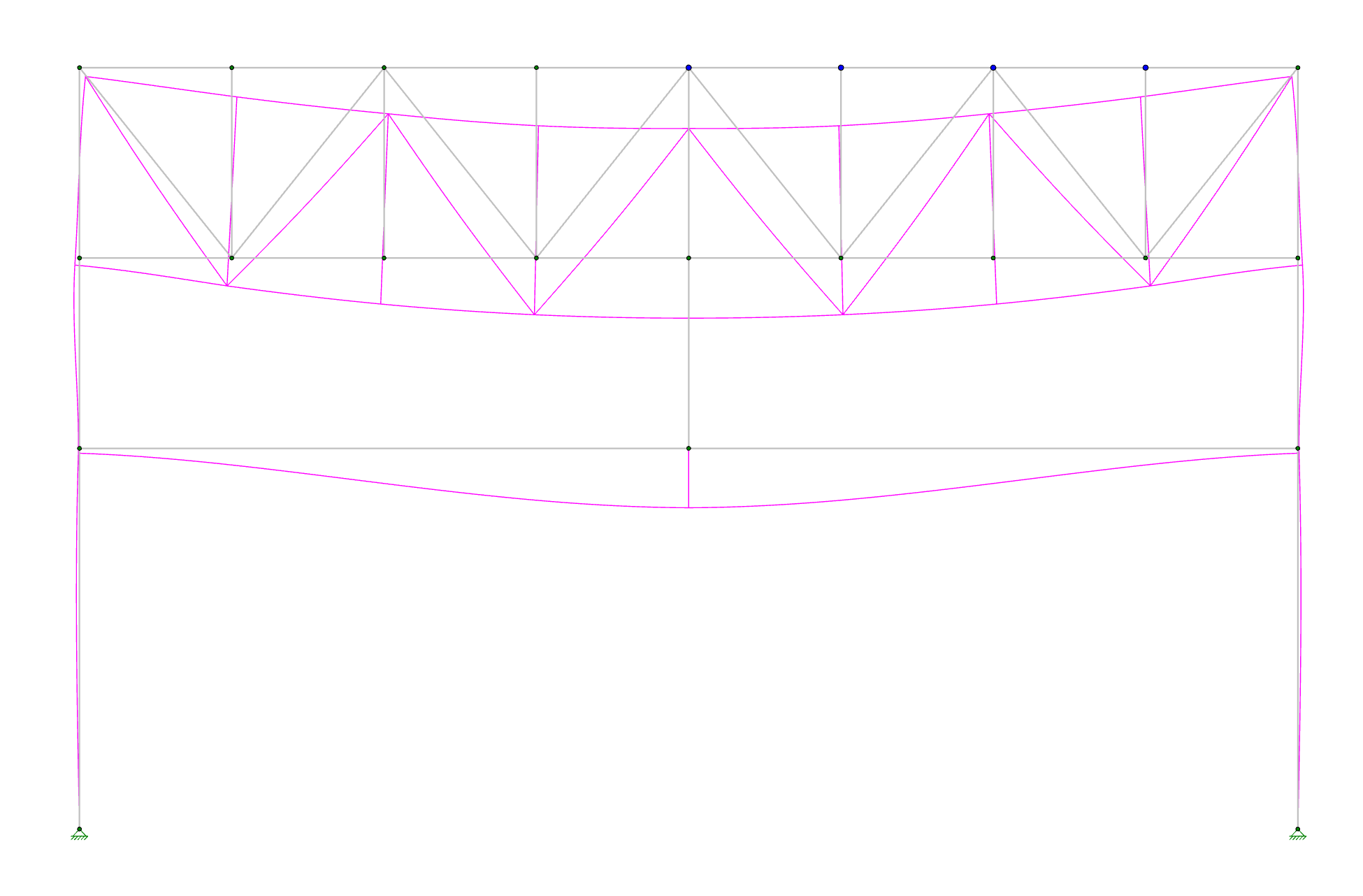
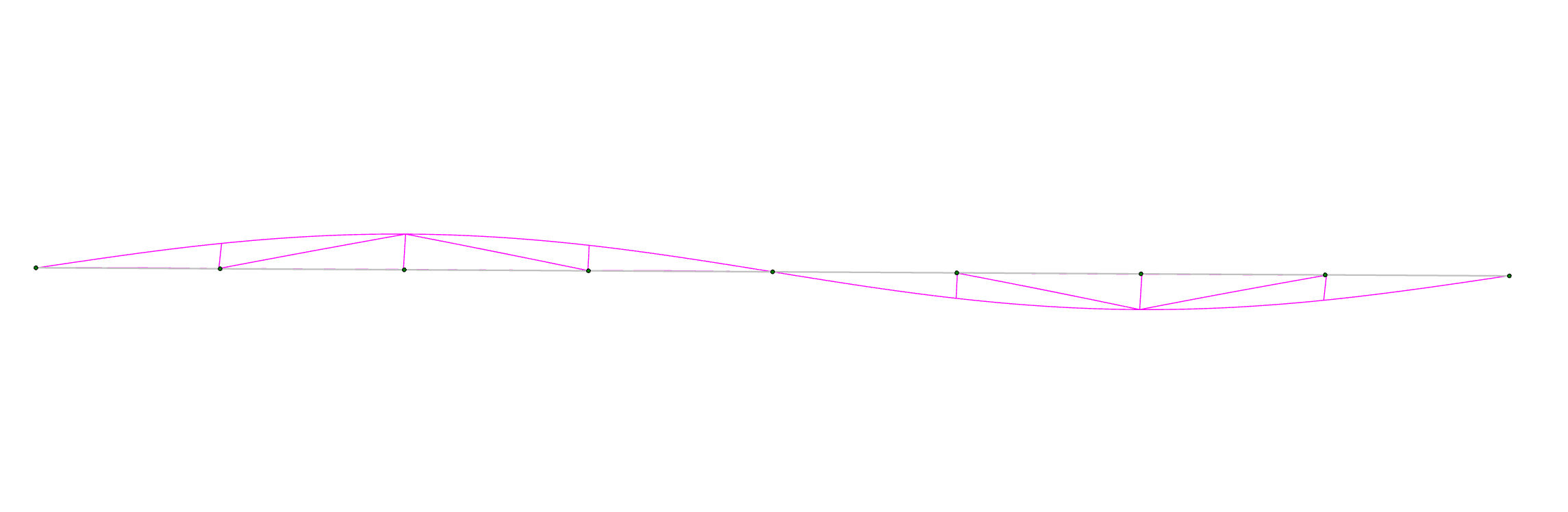
Once the buckled shape is understood, the solution is a relatively simple structural engineering decision. It may involve modeling some form of lateral restraint to mimic a roof diaphragm. Or, it may involve rotating the top chord so that the strong axis will resist this type of buckling.